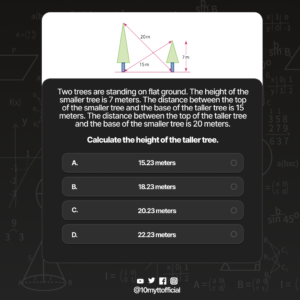
Question:
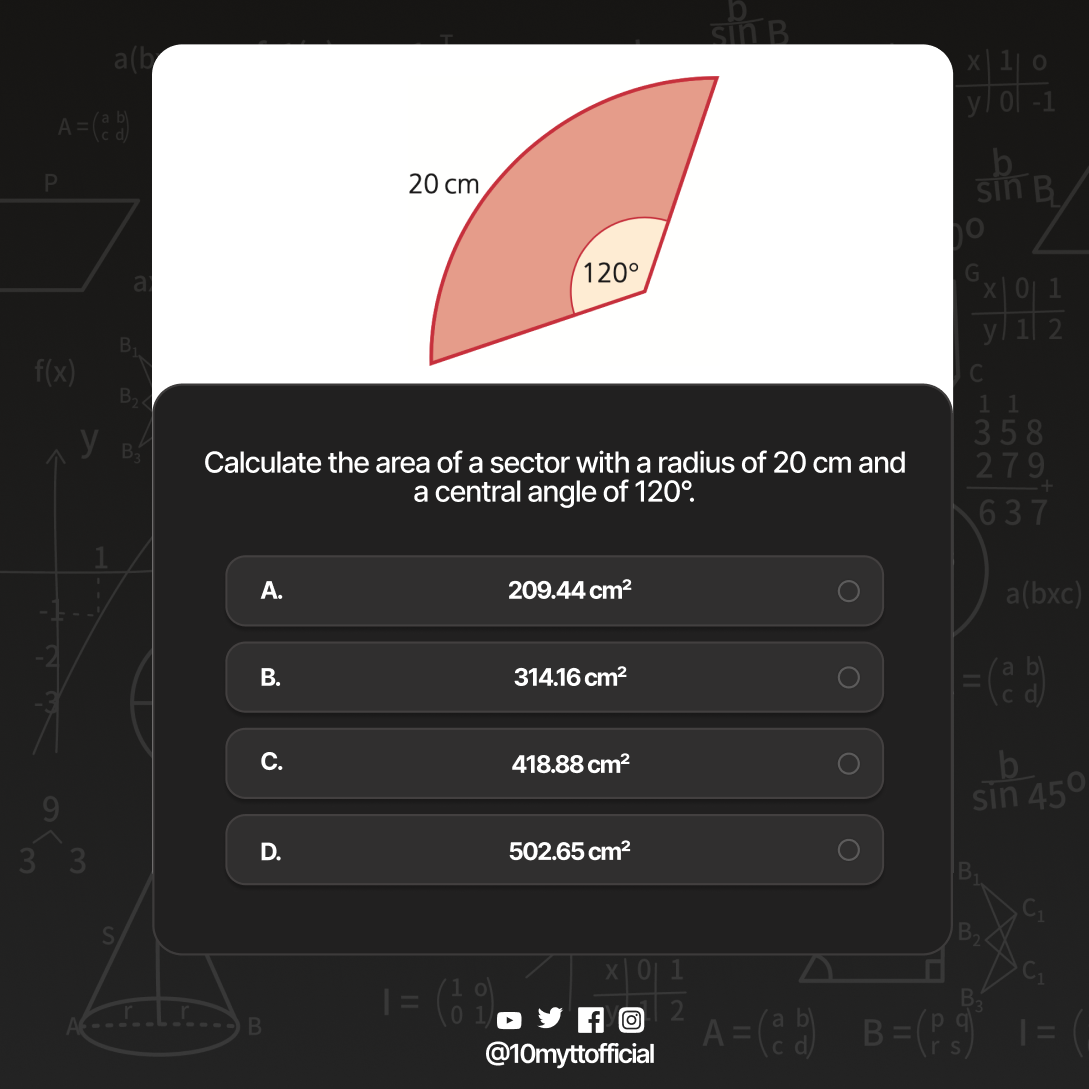
Calculate the area of a sector with a radius of 20 cm and a central angle of 120°.
A) 209.44 cm²
B) 314.16 cm²
C) 418.88 cm²
D) 502.65 cm²
Answer:
C) 418.88 cm²
To calculate the area of a sector, we can use the formula:
\[
\text{Area of Sector} = \frac{\theta}{360} \times \pi r^2
\]
where:
- \( \theta \) is the central angle of the sector in degrees,
- \( r \) is the radius of the circle.
Given:
- Central angle \( \theta = 120^\circ \)
- Radius \( r = 20 \, \text{cm} \)
Step-by-Step Calculation:
- Substitute the values into the formula:
\[
\text{Area of Sector} = \frac{120}{360} \times \pi \times (20)^2
\] - Simplify the fraction \( \frac{120}{360} = \frac{1}{3} \).
- Substitute and calculate the area.
The area of the sector is approximately \( 418.88 \, \text{cm}^2 \).