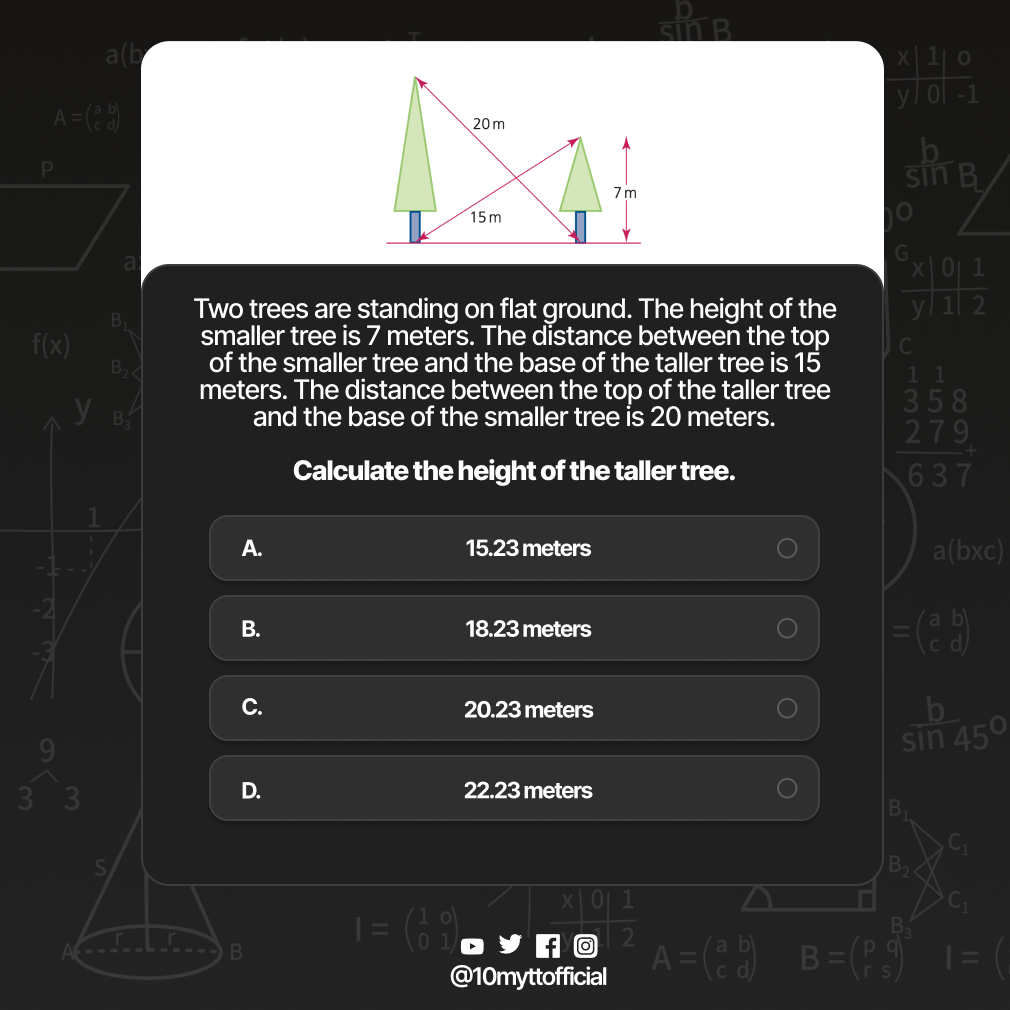
Question:
Two trees are standing on flat ground. The height of the smaller tree is 7 meters. The distance between the top of the smaller tree and the base of the taller tree is 15 meters. The distance between the top of the taller tree and the base of the smaller tree is 20 meters.
Calculate the height of the taller tree.
A) 15.23 meters
B) 18.23 meters
C) 20.23 meters
D) 22.23 meters
Answer:
C) 20.23 meters
Let’s solve this problem step-by-step:
Given:
- The height of the smaller tree = \( 7 \, \text{m} \)
- Distance between the top of the smaller tree and the base of the taller tree = \( 15 \, \text{m} \)
- Distance between the top of the taller tree and the base of the smaller tree = \( 20 \, \text{m} \)
Let \( h \) be the height of the taller tree.
Step 1: Set Up the Equation Using the Pythagorean Theorem
We have two right triangles formed by the heights and distances between the trees. We can focus on one of these right triangles to solve for \( h \).
For the right triangle formed by:
- The vertical side \( h – 7 \) (difference in height between the taller and smaller tree),
- The horizontal side \( 15 \),
- The hypotenuse \( 20 \).
Using the Pythagorean Theorem:
\[
(h – 7)^2 + 15^2 = 20^2
\]
Step 2: Expand and Simplify
- Expand \((h – 7)^2\):
\[
(h – 7)^2 = h^2 – 14h + 49
\] - Substitute into the equation:
\[
h^2 – 14h + 49 + 225 = 400
\] - Simplify:
\[
h^2 – 14h + 274 = 400
\] - Move 400 to the other side:
\[
h^2 – 14h – 126 = 0
\]
Step 3: Solve the Quadratic Equation
Now we have a quadratic equation in ( h ):
\[
h^2 – 14h – 126 = 0
\]
Using the quadratic formula:
\[
h = \frac{-(-14) \pm \sqrt{(-14)^2 – 4 \cdot 1 \cdot (-126)}}{2 \cdot 1}
\]
The solutions to the quadratic equation are:
- \( h = 20.23 \, \text{m} \)
- \( h = -6.23 \, \text{m} \) (which we discard because height cannot be negative)
Therefore, the height of the taller tree is approximately \( 20.23 \, \text{m} \).